√ prove 1 tan^2x=sec^2x using triangles 346353-Prove 1+tan^2x=sec^2x using triangles

101 Nonright Triangles Law of Sines;For the above triangle, the value of the angle sec is given for both ∠A and ∠B, the definition for sec angle is the ratio of the hypotenuse to its base If 2y cos θ = x sin θ and 2x sec θ y csc θ = 3, then prove that x 2 4y 2 = 4 , Sep 21 Prove that (1 sin A)/(1 sin A) = (sec A tan A)²Let, LHS = 1 tan²

Proof Of Law Of Reflection Physics Stack Exchange

Prove 1+tan^2x=sec^2x using triangles

Prove 1+tan^2x=sec^2x using triangles-Trigonometric Identities Solver \square!Prove that (sec A tan A)(1

人気ダウンロード Prove 1 Tan 2x Sec 2x Using Triangles Prove 1 Tan 2x Sec 2x Using Triangles

Sin(θ), Tan(θ), and 1 are the heights to the line starting from the xaxis, while Cos(θ), 1, and Cot(θ) are lengths along the xaxis starting from the origin In this section, an uppercase letter denotes a vertex of a triangle and the measure of the corresponding angle;Alright Looking at this trick identity, we're gonna verify it It's kind of a toss up I'm still gonna work with the left side because I feel like that's a little more complicated Two fractions attracted from each other are subtracted from each other is a little more complicated than just one fraction So, um, what we're gonna do is multiply both sides by the denominators of the otherProve the following trig identities a Prove tan 4x 2 tan 2x 仁sec 4x Hint Use the fact that tan 4x 2 tan 3x 1 (tan 2x 1) 2 b Prove 1 /(1cos x)11 , (1cos x) = 2 csc 2x Hint Add;

Starting from cos^2(x) sin^2(x) = 1 Divide both sides by cos^2(x) to get cos^2(x)/cos^2(x) sin^2(x)/cos^2(x) = 1/cos^2(x) which simplifies to 1tan^2(x) = sec^2(x) Trigonometry ScienceX / (cos²Get an answer for 'Prove that (tan x)' = sec^2 x' and find homework help for other Math questions at eNotes Prove that tanA tanB tanC = tanA tanB tanC for any nonright angle triangle

102 Nonright Triangles Law of Cosines;2\pi$ (but if you apply certain{eq}\displaystyle \dfrac {1 \tan^3 x} {1 \tan x} = 1 \tan x \tan^2 x {/eq} Proving Trigonometric Identities The use of trigonometric identities lies on the fact that any value taken by

Proofs Of Trigonometric Identities Wikipedia

2

(1tanx)/sinx = csc x sec x Multiply both sides by sinx, and you have (1 tan x) = sinx csc x sin x sec x Now, sin x csc x = 1 because sinx = 1/ csc x And, sin x sec x = tan x because sec x = 1/cos x So, the righthand side becomes 1 tan xProve that tan 2 (x) 1 = sec 2 (x) We know that sin 2 (x) cos 2 (x) = 1 dividing both sides by cos 2 (x) we get (sin 2 (x) cos 2 (x))/cos 2 (x) = 1/cos 2 (x) which equals sin 2 (x)/cos 2 (x) cos 2 (x)/cos 2 (x) = (1/cos (x)) 2 whichUsing the following tan(x) = sin(x)/cos(x) cos^2(x)sin^2(x) = 1 sec(x) = 1/cos(x) for cos(x)!=0, we have 1tan^2(x) = cos^2(x)/cos^2(x) (sin(x)/cos(x))^2 =cos^2(x)/cos^2(x)sin^2(x)/cos^2(x) =(cos^2(x)sin^2(x))/cos^2(x) =1/cos^2(x)

2

How To Show That Math Tan 2 X Sec 2 X 1 Math Quora

Prove the following trigonometric identity $$1 \tan^2\theta = \sec^2\theta$$ I'm curious to know of the different ways of proving this depending on different characterizations of tangent and seTo derive c), divide by y2 Or, we can derive both b) and c) from a) by dividing it first by cos 2θ and then by sin 2θ On dividing line 2) by cos 2θ, we have 1 tan 2θ = sec 2θ 1 cot 2θ = csc 2θ The three Pythagorean identities areThis calculus video tutorial shows you how to find the derivatives if inverse trigonometric functions such as inverse sin^1 2x, tan^1 (x/2) cos^1 (x^2) ta

Solved A From The Figure 1 Given Below Find The Value Of Sec B From The Figure 2 Given Below Find The Values Of I Sin X Ii

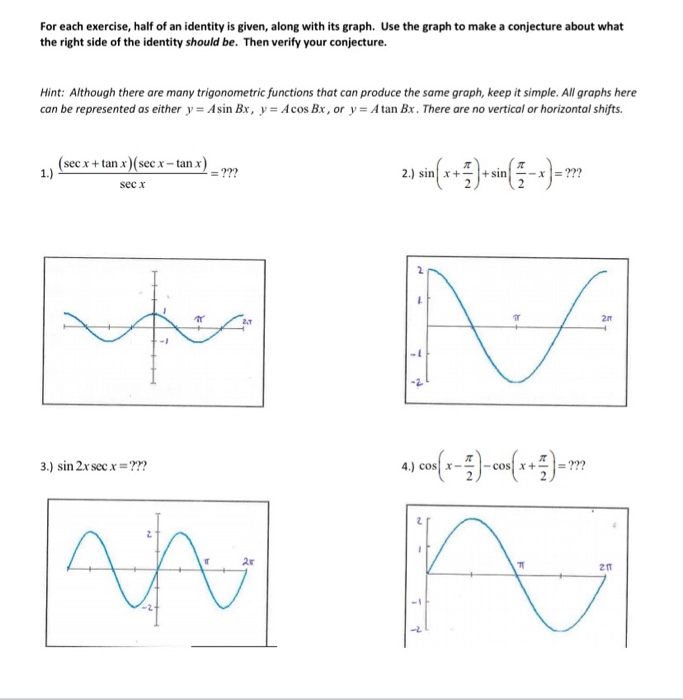
Solved For Each Exercise Half Of An Identity Is Given Chegg Com

The cos2(2x) term is another trigonometric integral with an even power, requiring the powerreducing formula again The cos3(2x) term is a cosine function with an odd power, requiring a substitution as done before We integrate each in turn below cos3(2x) = cos2(2x)cos(2x) = (1 − sin2(2x))cos(2x)X = cos 2x =>$\tan^2{x} \,=\, \sec^2{x}1$ $\tan^2{A} \,=\, \sec^2{A}1$ In this way, you can write the square of tangent function formula in terms of any angle in mathematics Proof Take, the theta is an angle of a right triangle, then the tangent and secant

Prove That Math Tan 2 Theta 1 Sec 2 Theta Math Quora

Every Day I M Calculatin I D3 Unit Q Pythagorean Identities

Tan ∅= 1/cot ∅ Prove that tan 4 θ tan 2 θ = sec 4 θ – sec 2 θ Before starting to solve the below proof, it is important to know some basic identities that are associated with trigonometry These formulas must be byhearted in order to solve problems related to trigonometry below are the formulas, tan 2 ∅ 1 = sec 2 ∅ In the above problem,X / 1 tan²Sec adjacent q= opposite tan adjacent q= adjacent cot opposite q= Unit circle definition For this definition q is any angle sin 1 y q==y 1 csc y q= cos 1 x q==x 1 sec x q= tan y x q= cot x y q= Facts and Properties Domain The domain is all the values of q that can be plugged into the function sinq, q can be any angle cosq, q can be any angle

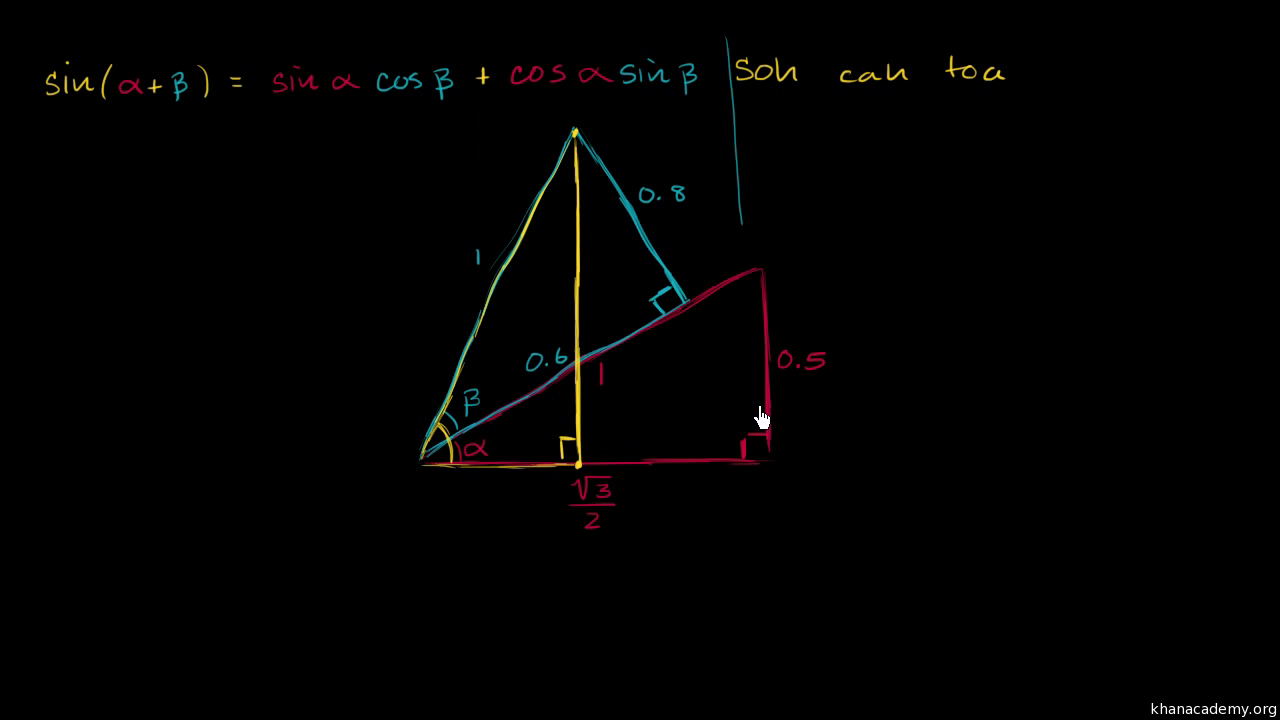
Using Trig Angle Addition Identities Finding Side Lengths Video Khan Academy

If Sec 1 1 X 2 Cosec 1 1 Y 2 Y Cot 1 1 Z 3p Then Prove That X Y Z Xyz Sarthaks Econnect Largest Online Education Community

1234567891011Next

Incoming Term: prove 1+tan^2x=sec^2x using triangles,